Create accountLogin

Recent searches

No recent searches

Search options

Only available when logged in.
mathstodon.xyz is one of the many independent Mastodon servers you can use to participate in the fediverse.
A Mastodon instance for maths people. We have LaTeX rendering in the web interface!

Administered by:

Server stats:

2.8K
active users

mathstodon.xyz: AboutStatusProfiles directoryPrivacy policy

Mastodon: AboutGet the appKeyboard shortcutsView source codev4.3.7

#QuantumFieldTheory

0 posts0 participants0 posts today
Public *
Bharath Krishnan

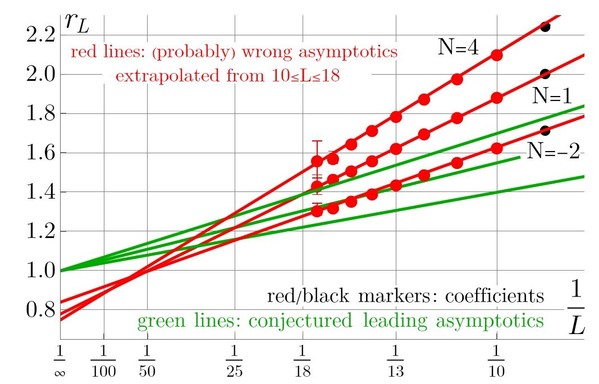
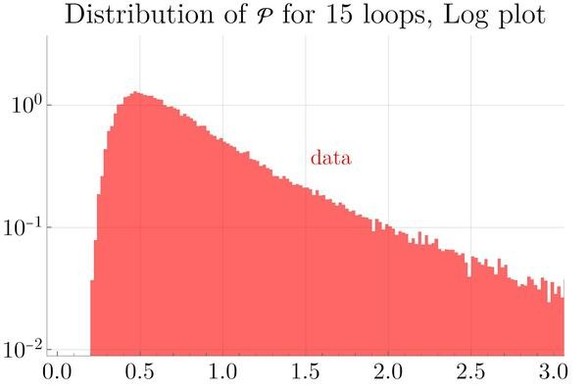
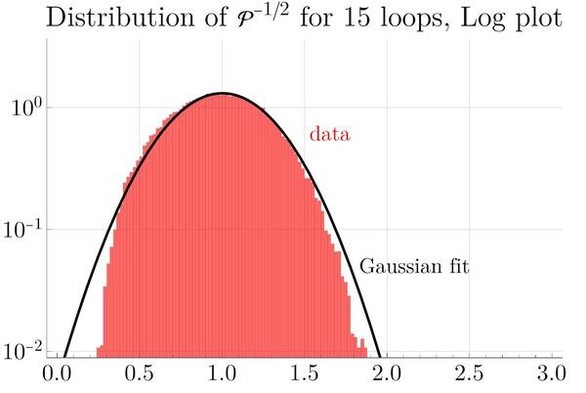
In a magazine article [1] on problems and progress in quantum field theory, Wood writes of Feynman path integrals, “No known mathematical procedure can meaningfully average an infinite number of objects covering an infinite expanse of space in general. The path integral is more of a physics philosophy than an exact mathematical recipe.”

This article [2] provides a method for averaging an arbitrary collection of objects; however, the average can be any number in the extension of the range of these objects. (Note, an arbitrary collection of these objects is a function.)

Question: Suppose anything meaningful has an application in quantum field theory. Is there a way of meaningfully choosing a unique, finite average of a function whose graph matches the description in Wood's quote?

For more info, see this post [3].

[1]: quantamagazine.org/mathematici

[2]: arxiv.org/pdf/2004.09103

[3]: math.stackexchange.com/q/50520

Quanta Magazine · Mathematicians Prove 2D Version of Quantum Gravity Really Works | Quanta MagazineIn three towering papers, a team of mathematicians has worked out the details of Liouville quantum field theory, a two-dimensional model of quantum gravity.
#PathIntegral#quantum#FeynmanPathIntegral
Public
Paul Balduf

New theoretical preprint arxiv.org/abs/2412.08617
We looked at the asymptotic growth rate of the beta function in , and the relative importance of subdivergence-free s. These graphs correspond to integrals, and the size of the graph is measured by its loop number, which also indicates how hard it is to solve the integral. State of the art computations in realistic theories are anywhere between 1 and 6 loops. The asymptotics of the perturbation series is known from instanton calculations. We now showed (in a model theory), that the leading asymptotics describes the true growth rate only for more than 25 loops, way beyond anything that can realistically be computed.

This is good news: It tells us that asymptotic instanton calculations provide non-trivial additional information that can not be trivially inferred from low-order perturbation theory.
In the plot, the red dots are numerical data points for the subdivergence-free graphs in phi^4 theory up to 18 loops, the green lines are the leading instanton asymptotics.

Public
MPI for Gravitational Physics

📣 Tiburtius Prize 2024 for Gustav Uhre Jakobsen 🏆

Recognition award for visiting postdoc at AEI Potsdam

Gustav Uhre Jakobsen, a postdoc at the Humboldt University of Berlin and in the Astrophysical and Cosmological Relativity Department at the @mpi_grav in the Potsdam Science Park, will be awarded a “Tirburtius Prize – Prize of the Berlin Universities” for his dissertation.

The reviewer praises not only the impressive wealth of topics in Jakobsen's doctoral thesis titled “Gravitational Scattering of Compact Bodies from Worldline Quantum Field Theory” and the quality of the research results, but also the impact it has had in the research community.

➡️ aei.mpg.de/1202051/tiburtius-p

www.aei.mpg.deTiburtius Prize 2024 for Gustav Uhre JakobsenGustav Uhre Jakobsen will be awarded the Tiburtius Prize 2024 for his outstanding dissertation on gravitational scattering of compact bodies from worldline quantum field theory. Learn more about the award and his research at the Max Planck Institute for Gravitational Physics and the Humboldt University of Berlin.
#ResearchAward#Berlin#PhDThesis
Public
Petri Laarne

🚨 New !

We study a stochastic PDE whose solutions want to be close to constant -1 or +1. But because it’s stochastic, the solutions occasionally jump between those two optima. How often, on average?

In technical terms, we study a certain nonlinear wave equation whose invariant measure is the ϕ4 . The average transition time is called an Eyring–Kramers law, asymptotic in the low-temperature limit. It has already been derived for 2D stochastic heat equation and 1D wave; we extend it to 2D and 3D wave equations.

Joint work with my PhD advisor Nikolay Barashkov.

arxiv.org/abs/2410.03495

arXiv.orgEyring--Kramers law for the hyperbolic $ϕ^4$ modelWe study the expected transition frequency between the two metastable states of a stochastic wave equation with double-well potential. By transition state theory, the frequency factorizes into two components: one depends only on the invariant measure, given by the $ϕ^4_d$ quantum field theory, and the other takes the dynamics into account. We compute the first component with the variational approach to stochastic quantization when $d = 2, 3$. For the two-dimensional equation with random data but no stochastic forcing, we also compute the transmission coefficient.
Public
Paul Balduf

Two years ago, I began writing my in theoretical . Most effort went into giving a very detailed pedagogical account of what the in does, and why it is natural and transparent from a physical perspective.
One year ago, my referees recommended in their reports to publish the thesis as a book, and today I received the printed copies!
It was exciting to go through all the steps of actually publishing a book, and I hope that it will be of use to convince physicists that the Hopf algebra structure in is not a weird mathematical conundrum, but it actually encodes the very way physicists have been thinking of renormalization since the 1950s: Parametrize a theory by quantities one can actually measure, instead of fictional expansion parameters.
link.springer.com/book/10.1007

Public
Paul Balduf

In , scattering amplitudes can be computed as sums of (very many) s. They contribute differently much, with most integrals contributing near the average (scaled to 1.0 in the plots), but a "long tail" of integrals that are larger by a significant factor.
We looked at patterns in these distributions, and one particularly striking one is that if instead of the Feynman integral P itself, you consider 1 divided by root of P, the distribution is almost Gaussian! To my knowledge, this is the first time anything like this has been observed. We only looked at one quantum field theory, the "phi^4 theory in 4 dimensions". It would be interesting to see if this is coincidence for this particular theory and class of Feynman integrals, or if it persists universally.
More background and relevant papers at paulbalduf.com/research/statis

Public
Vanessa

I am currently investigating an analogy between geodesic deviation from GR and the electromagnetic (Lorentz + Coulomb) force in QED. Once I got all the mathematical details worked out, I will make a thread about this, but I am tripping over the details of how to solve the classical Dirac equation perturbatively in a constant external EM field (mainly distribution-theoretical Fourier stuff). Does anyone here have a good reference on this?

#physics#theoreticalphysics#quantumfieldtheory
Public
Erwin Schrödinger Institute

The newest publications following the thematic programme on at the Frontiers of the tell us that August was a productive month at
@ESIVienna. 🤩

Check out one of the articles released just now:
Bahman Dehnadi, André H. Hoang, Oliver L. Jin, Vicent Mateu / Top Quark Mass Calibration for - An Update

arxiv.org/pdf/2309.00547.pdf


@univienna

Public *
Erwin Schrödinger Institute

We are happy to announce that a new thematic programme with 5 focus weeks just got started at
@ESIVienna
! 🥳 Check out the details below ⏬

📅 31st July - 1st September 2023 📅
📌 Schrödinger Lecture Hall 📌

▫️ Programme description ▫️
esi.ac.at/events/e476/

📚 Subject: at the Frontiers of the
📓Week 1: Finite-Mass and Effects in
📕 Week 2: Structure of Quantum Field Theory Beyond the Leading Power
📗 Week 3: Violation and the of Universal Functions
📘 Week 4: Simulation of the All Order Structure of Scattering Amplitudes
📙 Week 5: Multi-Variable Techniques for All Order Resummations in QFT

(see the motion picture at twitter.com/ESIVienna/status/1)

@univienna

www.esi.ac.atActivitiesThe Erwin Schroedinger International Institute For Mathematics and Physics
Public *
katch wreck

"We show that optical bias fields injected into multistable #optical systems enable a controllable source of #quantum #randomness, and we demonstrated this concept in an optical parametric oscillator (OPO). By injecting bias pulses with less than one #photon on average, we controlled the probabilities of the two possible OPO output states."

science.org/doi/10.1126/scienc

#quantumFieldTheory#fieldTheory#fluctuations
SearchLive feeds
About