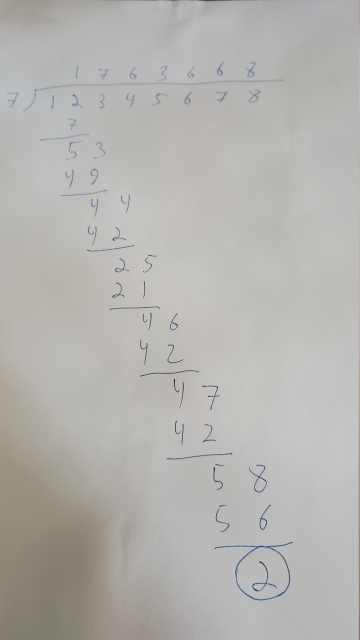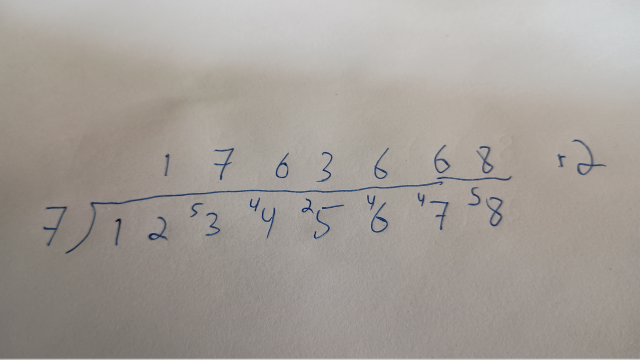Back

Another handy trick is, when checking if 509 is a multiple of 19, you do *not* start by dividing 509 by 19 getting 380 plus some yucky remainder. Instead you observe that 509 = 19 + 490 and 49 is not a multiple of 19 so you move on.

I only thought of this embarrassingly late in life.

An elaboration on this is that when factoring smallish numbers of the form $n=10k+1$ you will often need to test for divisibility by 7 and then by 13 in succession. But $7\cdot 13=91$ so instead of considering $n$, consider $$𝑛-91=10k-90=10\cdot (k-9)$$ and do both at once.

For example, instead of dividing $5031$ by $7$ and then by $13$, consider $503-9=494$ instead. This is evidently not divisible by $7$, but for $13$ you can see from $494+26=520$ that it is a multiple of $13$, and therefore so too was $5031$.

I'm talking about mental calculation here, or maybe abacus. For computer calculation it's obviously stupid.

My kids' schools never taught them short division, which seems to me a sad and odd omission.

The idea is that instead of doing the big hairy thing on the left you do the compact speedy thing on the right. The left-side technique should only be used when the divisor is bigger than the multiplication tables you have memorized.

The thing on the right is simple enough to do in your head, and particularly so if you only need the remainder and not the quotient. My kids were often mystified by my ability to divide in my head but it's just that I was taught a better algorithm than they were.

I blogged about this a while back as part of a longer article about several methods of checking for divisibility by 7: https://blog.plover.com/math/divisibility-by-7.html

@icecolbeveridge I really need to start doing this, thanks.

@svat Yes, for 6 years I have been too lazy to correct the picture, because the article is about divisibility testing, and the wrong quotient doesn't affect that.

@mjd It's not just about the quotient — I had never encountered the term "short division" before (we were only taught "division" which is closer to the picture on the left, but in practice I was doing something more or less like the picture on the right) so I was trying to understand "short division" using the picture, so I didn't understand how you got the 4 or the final 9 in the quotient, or even the remainder of 9 instead of 2 (which is equivalent yes but it was confusing). Now I understand what was intended though :)

@svat Yes, I really should fix it, it detracts from the article.

@icecolbeveridge Sorry, I answered the wrong question before.

My trial division by $13$ has revealed that $509=13\cdot 30+119$. I know that $119$ is a multiple of $17$ and that $13\cdot 30$ isn't, so their sum can't be.

I know that $119$ is a multiple of $17$ because I happen to remember that $119=7\cdot 17$. And obviously $17$ can't divide $13\cdot 30$ because it would have to divide $13$ or $30$.

@mjd ok, good, we're on the same page :-)

@jhertzli I don't understand how that helps. Please elaborate.