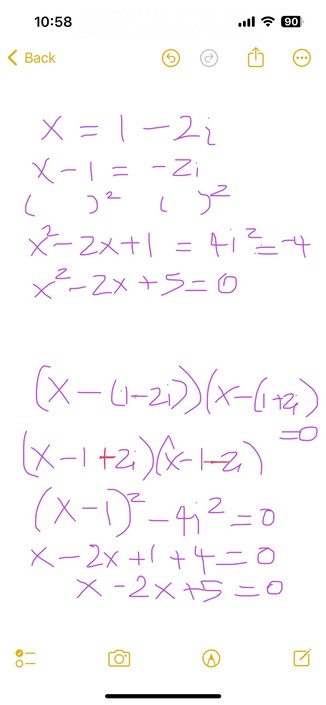Today's highlight from the Back-To-School Tour of the TI84 is Function Fundamentals: TI84 Tips for #Algebra2

How to call on function definitions for easier calculations & graphing!

#MTBoS #iTeachMath #T3Learns #ClassroomMath #MathEd #MathsEdChat #TI84 @TICalculators

https://karendcampe.wordpress.com/2021/08/29/function-fundamentals/

Happy Saturday! Let's do some quick #ProblemSolving using #TryMathLive #TryMathsLive from the February Calendar of Problems.
Here is Feb 9, a great one to remind Ss of exponent laws & common base strategy.
#Algebra2 #Precalculus #MTBoS #iTeachMath #ClassroomMath #MathEd #MathsEd

Open box problem in #Algebra2 today. Students made a variety of open boxes by folding up index cards where each square corner (x by x) has been cut away. What should x be to produce a box of maximum volume?

I had students look at their boxes and make a guess. It was interesting to hear their reasoning. Afterwards, they measured all the volumes, and we learned that most students guessed wrong.

We then found the polynomials for the volume of our box, graphed it and our data and realized both looked kind of quadratic in the domain we were using. Had students zoom out to discover what the functions actually looks like. This is the first time they have seen a graph of a cubic, so they were pretty impressed by it.

More discussion ensued about a proper domain and range for our data set and how that related to the graph. Students left feeling saturated with math.

Hi there @DavidKButler the Operations Tower made an appearance in #algebra2 tutoring yesterday!
To explain why (7 + x)^2 ≠ 49 + x^2
Thanks!!
#ClassroomMath #MTBoS #iTeachMath #MathEd #MathsEd

If your students know nothing of vectors, how would you introduce multiplying matrices? I have always done dot products as a lead in to matrix multiplication but that is not an option this year. How can I make multiplying all those rows and columns make sense to a student without that background?

Introducing systems today. Had the students make 2 lists; one where pairs of numbers summed to 6, and the other with pairs of numbers whose difference is 11. Then asked them to find a pair of numbers that are on both lists.

Absolute crickets.

I had to ask leading questions, give hints, ask them if they had tried such and such. I really had to drag them along before they realized they could do something other than stare at their paper.

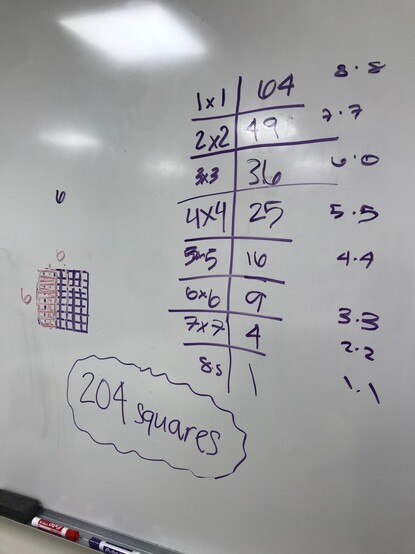
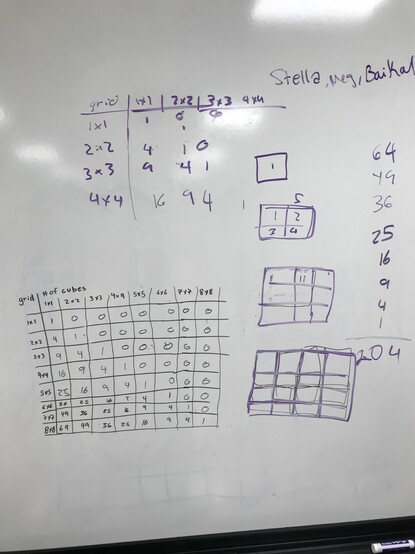
So the next period I had them start standing at the whiteboards before I gave them this task. And I did not have to seed them at all. Some were graphing the pairs and realized they made lines. Others were finding the equations of the lines and realizing they needed to find the intersection point. All in all, in 15 minutes I watched the whole class figure out systems of equations. I taught them nothing.

I really feel like when the students are sitting in their desks, they are waiting for me to give out answers and pour knowledge into their brain. But when they are standing at the whiteboards, they take control of their learning. They are willing to try different things, take risks. And they don't wait for me to tell them what to do.

#ThinkingClassroom for the win.

It's been a long week. We lost a student from our school over the labor day weekend and the atmosphere has been low. Time for a light hearted activity to end the week.

Inspired by Fawn Nguyen's "Vroom Vroom" post on her blog.
https://www.fawnnguyen.com/teach/vroom-vroom

Students measured the pullback distance of a car and then measured the distance it rolled. Graphed the data. Found the line of best fit by eye, then found the linear regression. Made some predictions, calculated some residuals. Summed up all the stuff we've been learning the past week.

I then picked a random distance and groups calculated their pullback distance. Everyone lined up their cars that distance from the finish line, pulled back their calculated distance, and let 'em rip. The group whose car got the closest to the "finish line" (marked by tape on the floor) got bragging rights.

Needed an extra lesson on lines in #Algebra2 since one class met an extra period this week for some unknown reason. Bouncing ping pong balls to the rescue! Students dropped ping pong balls from different heights and measured the rebound height, plotted the data and found a linear model. We haven't learned about regressions yet (soon though!) so they used a ruler to eyeball the best line.

Challenge time. I took all the balls away and told them to use their model to calculate the proper drop height for the ball to rebound to 78 cm. I rigged up a small hoop I had lying around at just that height so everyone in the class could tell whether the rebound was to the right height. Hilarity ensued when several groups realized their drop height predictions were below the rebound height. Turns out they plugged in for x when they should have plugged in for y. A good learning moment.

It was a pretty basic, last minute lesson, but I think it went ok. It was nice having everyone's data, graph, and model on the whiteboards around the room for all to see. I have whiteboards on all 4 walls (no windows though!).

Found a new way to introduce, and review linear equations in #Algebra2 today. I had students give me 2 numbers, and we added them together to get a third. Then we added the previous two to get a fourth and so on (3,7,10,17,27,...). The task was for the students to find what the first two numbers should be such that the 5th number in the sequence is 100. So they went to work.

The students found lots of possible solutions. Some of them were very organized, most were not. Students who organized their work found lots of patterns. We all learned from each other. Then I had them graph all their solutions (first number on the x-axis and second number on the y axis.) The solutions made a line! What? We noticed how the slope of the line could be seen in their table of data. Then they set out to find the equation.

By the end of class we had reviewed slope-intercept and standard form of linear equations, found x and y intercepts, and reviewed how first differences in a table of numbers can show you the slope. It was a good day.

Day two in #Algebra2 went well. Did the checkerboard problem (Counting the number of squares in an 8x8 checkerboard, look for patterns, extend to an nxn board). Yesterday we experienced how using tables to organize our thinking could be useful and illuminate unforseen patterns, so I was happy to see many groups using tables today. I was especially giddy when two groups saw they had different numbers in their tables, but they added up to the same total, and then proceeded to spend some time trying to understand eachother's approach. All in all, a good day.

Finished my syllabi today for my 3 classes. I'm teaching #algebra2, Precalculus, and AP #Calculus. I'm hoping I am not out of my depth. Though I believe it will be easier than the Algebra 1 classes I had last year, as those were all Freshmen and this year should be mostly Seniors. At least I feel a little bit better having gotten an idea of my classroom procedures and how they are changing from last year.

So, I need some input from other #teachers in #education who are #teaching on a #blockschedule. This is the first time that my school will be doing it, this is only my 2nd year teaching and last year we had normal 50-minute classes. I am going to be teaching #algebra2, #precalculus, and #apcalculus. My question is -- what break duration do you suggest? I'm thinking 5 minutes and give them the chance to walk around the room and such, get up, stretch, at about 40 after class starts, pick up again from 45 after and continue until the 90 minutes is up. I would love to get input?

Hello #mtbos . Been offline the past year. Excited to get back to learning from everyone and sharing my journey as #iteachmath . I have taught it all over the past 20+ years and will be teaching #Algebra2 and Multivariable and Vector Calculus this up and coming year. I’ve been trying to build a #thinkingclassroom the past 2 years and am always on the lookout for good, rich, spiraling tasks.

Any #Algebra2/#Precalc level students out there? I need student helper beta testers. This is a mystery picture graphing project, where the points to be plotted are solutions to #SystemsOfEquations, and students are directed to use their choice of two #matrix methods.

https://app.teachermade.com/begin/b5679ec9-15ad-4d5c-9e11-92480b38327c

I will add tutorial videos on the solution methods to the final version — I will be happy to give someone a 1:1 help session, with their consent to record and use it.