6/
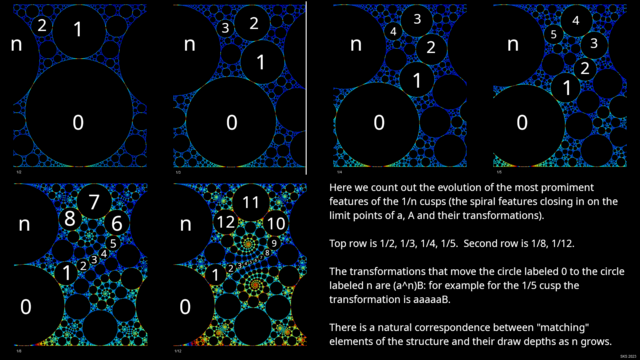
In general, the smoothly-connecting double cusp groups with numerator k are clustered into groups where the denominator is of the form
So, for example:
- if k=4, m ϵ {1,3}
- if k=5, m ϵ {1,2,3,4}
- if k=6, m ϵ {1,5}
- if k=7, m ϵ {1,2,3,4,5,6}
- if k=8, m ϵ {1,3,5,7}
There are always an even number of such sub-streams, and they come in pairs (where m=j, k-j) where the structure is similar, but complementary. For example, when k=7, the paired streams are {7n+1, 7n+6}, {7n+2, 7n+5}, {7n+3, 7n+4}.
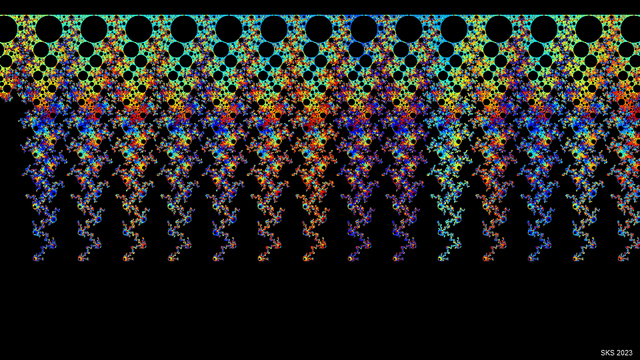
Attached are several movies that show this effect when the numerator is 7.
- the first one, titled "7n-Smoosh", shows what a concatenation looks like that includes all denominators in order, with maximum denominator 150
- the other three are titled "7n+1", "7n+2", and "7n+3" with maximum denominator 250
As was the case for the case where the numerator is 3, the relative beauty of these videos is in the eye of the beholder, but the ones that are constrained to constant offsets of a multiple of the numerator are much more consistent to one another.