Create accountLogin

Recent searches

No recent searches

Search options

Only available when logged in.
mathstodon.xyz is one of the many independent Mastodon servers you can use to participate in the fediverse.
A Mastodon instance for maths people. We have LaTeX rendering in the web interface!

Administered by:

Server stats:

3K
active users

mathstodon.xyz: AboutStatusProfiles directoryPrivacy policy

Mastodon: AboutGet the appKeyboard shortcutsView source codev4.3.7

Patrick Honner

The new year is one of my favorite kinds of numbers: a difference of squares!

2024=(45+1)×(45−1)=452−12

This observation got me thinking about what kinds of numbers can be written as the difference of squares. For example, 3=22−12,5=32−22, and 16=42−02, but it is impossible to write 6 as the difference of squares of integers.

So here’s a little mathematical puzzle to start the new year: Is there a largest number that can not be expressed as the difference of squares? If so, find it. If not, prove no such number exists. Good luck, and happy new year!

mrhonner.com/archives/21614

#2024

Mr Honner · 2024 and Differences of SquaresThe new year is one of my favorite kinds of numbers: a difference of squares! $latex 2024 = 46 \times 44 = (45 + 1) \times (45 – 1) = 45^2 – 1^2 $ This observation got me thinking about…
Jan 01, 2024, 02:40 PM·Public·Web
3boosts·6favorites
Public
Dave Neary

@phonner A hint: consider the parity of a−b and a+b.

Public
Karen Campe

@phonner Hi Patrick!
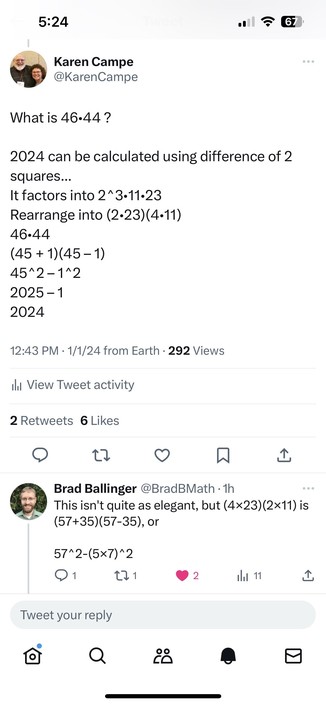
I had noticed the difference of 2 squares opportunity for 2024, and it turns out it can done TWO ways:
45^2 – 1^2
and
57^2 – 35^2

Screenshot of twitter posts: Karen noticed 2024 factors to 2^3•11•23 Rearrange into (2•23)(4•11) 46•44 (45 + 1)(45 – 1) 45^2 – 1^2 Brad noticed 4×23)(2×11) is (57+35)(57-35), or 57^2-(5×7)^2
Public
Patrick Honner

@davidradcliffe @KarenCampe Figuring out *when* it's possible also allowd you to determine in how many different ways it's possible.

Public
Karen Campe

@phonner @davidradcliffe oooh, I am excited to figure this out!

Screenshot of GIF rubbing hands together in anticipation
Public
Karen Campe

@phonner @davidradcliffe
So I need both factors of 2024 to be even (or both odd, which won't happen with prime factorization 2^3•11•23
Spoiler ahead....

Public
Karen Campe
Public
Bruce

@KarenCampe @phonner Actually 2024 can be written as a difference of squares in four ways: not only 45^2 - 1^2 and 57^2 - 35^2, but also 507^2 - 505^2, and 255^2 - 251^2. (Hint: write 2024 as the product of two even numbers; one factor will be a+b and the other a-b.)

Public
Karen Campe

@byoshiwara YES, if you keep reading down that thread, I figured out the four ways...
What a fun math-y excursion!

Public
Ben

@phonner you may be interested in a video I made a couple of years ago where I make a sieve out of the difference of squares. youtu.be/22SN8otf4iI?si=ucav0V

One of the follow up videos was about which even numbers can be expressed as the difference of two squares in only one way. youtu.be/aDBTNEPqkZk?si=pr7m8S

I also worked out how to optimise which square numbers you need to factorise any semi-prime number. Between trial division and the bounds created in the first video above combined with this optimisation you can infinitely narrow down the factors of any potential prime (except 2mod4 numbers). youtu.be/K5qplL_ahTo?si=tNIeD7

YouTubeA different perspective on the distribution of prime numbersThe Sieve of Eratosthenes is an amazing tool for teaching people about prime numbers and composite numbers but it's not without its limitations.This is my at...
Public
Akshar Varma
SearchLive feeds
About