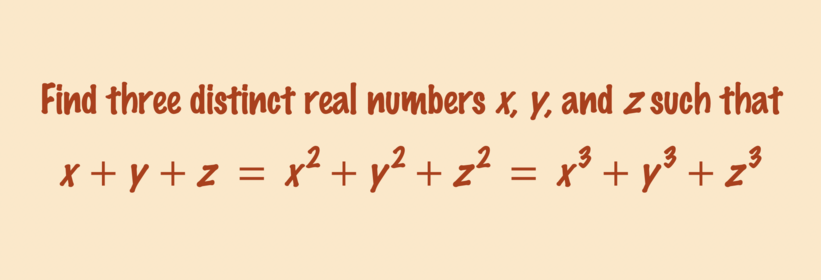Create accountLogin

Recent searches

No recent searches

Search options

Only available when logged in.
mathstodon.xyz is one of the many independent Mastodon servers you can use to participate in the fediverse.
A Mastodon instance for maths people. We have LaTeX rendering in the web interface!

Administered by:

Server stats:

3K
active users

mathstodon.xyz: AboutStatusProfiles directoryPrivacy policy

Mastodon: AboutGet the appKeyboard shortcutsView source codev4.3.7

Public *
Matt Enlow

@futurebird The sum of their squares is equal to the sum of their cubes, but I don't think they are equal to their sum.

Public
myrmepropagandist

@UnsolvedMrE
You just need any point on y**2 + z**2 = y**3 + z**3 where yz!=0, y!=z right?

Public *
Jan Van lent
Public *
Jan Van lent

@UnsolvedMrE Method:
- observe that the first equation describes a sphere
- translate coordinates so that the sphere is centered at the origin (e.g., by completing squares)
- rotate coordinates so that the x+y+z=1 plane becomes orthogonal to the z axis
- introduce spherical coordinates (theta, phi)
- rearrange curve equation for phi
- substitute back and reverse the coordinate transformations

There are probably quicker ways to get to the same answer, but this was my process.

Public *
Jan Van lent

@UnsolvedMrE Update: I had the phase angles wrong. Should be 2*pi/3 and 4*pi/3, instead of pi/3 and 2*pi/3.

Public
Pellouze

@UnsolvedMrE nice one! Haven't found it yet, so far I can prove x y z are not in {0,1}, and they're not equally spaced (aka they're not a, a-b, a+b)
Other than that, still no clue and I need to get back to work

SearchLive feeds
About