Back

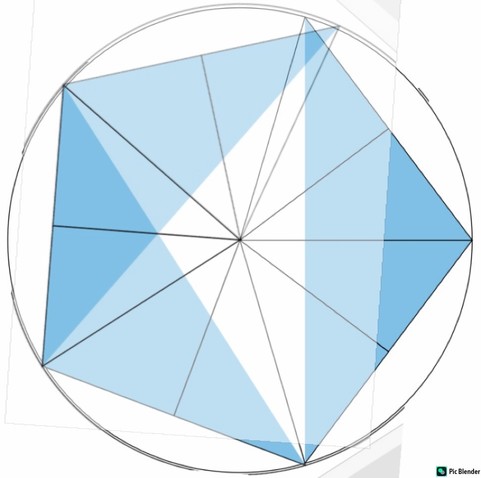
@11011110 Tom Kelly here. I am trying to wrap my head around your post. To simplify the question, I make the radius 5. The sides of the four small right triangles are rational, namely 3 and 4. What are lengths of the sides of the larger blue triangles in this case? They seem to be somewhat smaller than 3-4-5. Do they have an integer value? A fractional value? Thank you.

@TomKellyTAK If you scale my second illustration so that the circle has radius 5 and the four right triangles in it have sides 3-4-5, then the two sides of the blue isosceles triangle are 6. It can be bisected into two right triangles similar to the 3-4-5 right triangles (they have the same angle as the 3-4-5 at the apex of the blue isosceles triangle, and right triangles with one equal non-right angle are similar), with hypotenuse 6, so the longer of the two other sides of these right triangles is 6(4/5)=24/5. The base of the blue isosceles triangle is twice that, 48/5.

@11011110 thank you. Just recently saw your answer by accident. I will have to figure out mastodon. I am at work, but To rephrase, if the radius is 25, then the four right triangles are 15,20,25. The two blue triangles are 18, 24, 30. The final combined blue triangle has a base of 48. As I understand your project, The next step in making a cluster of points on the circle is to rotate the large blue triangle. Roughly as below. Or perhaps to rotate the blue triangle in small steps. Am I following you correctly?

@TomKellyTAK Yes. You can rotate as many times as you want and the distances will stay rational. But if you rotate more times you are going to need larger scale factors to keep the distances integral.

@11011110 I assume you are interested in making a cloud of points such that they essentially “form” a circle. In that case, the legs of the Pythagorean triplets would have to be very large, in the thousands and millions to keep the lengths integer. I believe someone mentioned using a tangent function to find Pythagorean triplets close to a certain degree. To put the triplets in order by degree or minutes seems difficult and haphazard. Are there any other patterns you have noticed besides the even number rule you found? Thanks for the help. Will post my drawings soon.

@TomKellyTAK I don't care about the circle shape in this case; what I care about is integer distances and non-collinearity. But the only way we know to get large non-collinear integer-distance sets is to put them on circles.