Recent searches
Search options
Happy 2025!
There's some lovely numerology here. 2025 is 1³+2³+3³+...+9³ . And 2025 is also 45² , which is (1+2+3+...+9)². Some of my best friends are squares of triangle numbers!
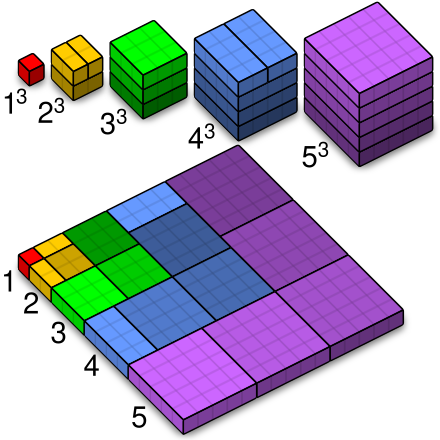
This isn't just a coincidence: In general, 1³+2³+3³+...+𝑛³=(1+2+3+...+𝑛)²
There's a lovely proof-without-words picture of this. Heh — I just found out that in fact it's so good that it is the top picture in the Wikipedia page https://en.wikipedia.org/wiki/Proof_without_words
This picture shows what happens with 𝑛=5, but you can do the same thing for any n. (Just notice that even n's get the top layer of the cube cut in half while odd n's keep all their layers whole.)
(1/3)
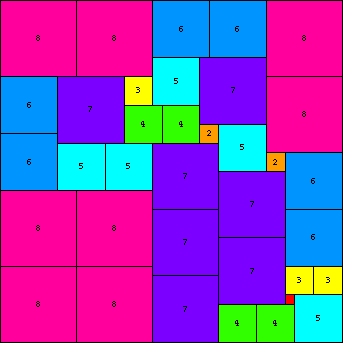
This also happens to be the identity underlying the "Partridge problem". While the proof-without-words uses three dimensions to get the cubes, you can also represent 𝑛³ in two dimensions by using 𝑛 copies of a thing of size 𝑛². For 𝑛=8, you can use one 1×1 square, two 2×2 squares, three 3×3 squares,..., and eight 8×8 squares to cover one square of edge length 1+2+...+8, as pictured here.
Finding this was called the "Partridge problem" not because it was proposed by, like, some guy named John Partridge but rather because "4 calling birds, 3 french hens, 2 turtle doves, and a partridge in a square tree." This picture, and many more, come from Erich Friedman's page about it, https://erich-friedman.github.io/mathmagic/0802.html.
It's totally not obvious that this kind of tiling is even possible, and it doesn't work for squares with 𝑛<8, and unlike the proof-without-words version, there is nothing here that makes it clear that the side-length of the square is 1+2+3+...+8. But it's neat that this decomposition of a square into smaller squares is possible at all.
(2/3)
Moreover, the area of *any* shape in the plane increases by a factor of 𝑛² when you scale it up by a factor of n. (Welcome to what "two dimensional" really means, or maybe what "area" means.) So this identity about numerical squares and cubes lets you try to play tiling games with any planar geometric shape. For 2025 specifically, we want something with n=9, and that is delightfully the equilateral triangle.
The big triangle has area 2025 times the area of the small red triangle. Happy New Year.
(3/3)
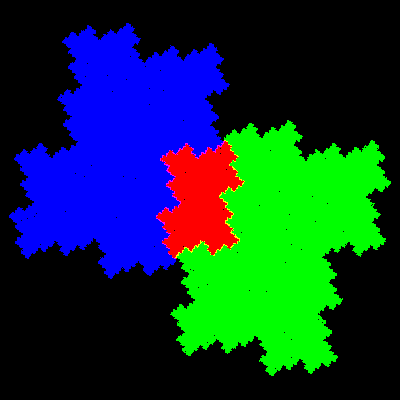
Okay okay actually just one more thing. My *favorite* open question about the Partridge problem is this: Is there *any* planar shape at all which solves it with 𝑛=2??
That is, is there any planar shape P where one copy of P and two copies of double-sized P can be fit together to make a triple-sized P? The areas work out, since 1+4+4=9, that same identity again.
Last year I briefly thought I found a P that worked! Here's a picture. But the blue and green ones are sadly *not* 2/3-scale copies of the overall picture; instead the scaling factor 𝑠 is the positive root of 𝑠⁶+2𝑠²=1, around 0.673348. Whomp whomp.
If you want a delightful puzzle to try yourself: There is a Partridge tiling with 𝑛=4 of the 30-60-90 triangle! I won't post a picture here, in case you want to cut out ten 30-60-90s of your own (of sizes 1,2,2,3,3,3,4,4,4,4), and try to assemble them into one big one (of size 10).
(4/3, ok really done this time, I am bad at counting or self-control or predicting the future or something)
@Log3overLog2 happy 9 year!
2+0+2+5 = 9, and 2025/9 = 225, and 2+2+5 = 9
@sirjofri Yes indeed! 2025 is 45² and 45 is 5×9, so 2025 is chock full of 5's and 9's coming and going.
@Log3overLog2 cirno and glenda are very happy, I guess