Back

every vector has a basis is implied by
#calculus

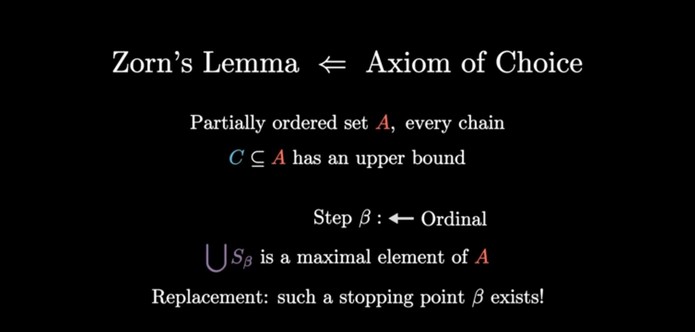
@xameer I think all three are equivalent but the proof I learned in functional analysis was via Zorn.

@jayalane naturally well ordered? what does that even mean?

@xameer well ordered by the natural order <. every subset of the natural numbers, or positive integers, has a least element by the normal total order of <. The integers does not have that property. So the integers aren’t well ordered by <

@jayalane natural well order : R( normal total order)
without specifically defining normal this is some variant of circular explanation
to explain natural you use normal , without clarifying what re both and how do they relate in general.
I mean before you extend the loop further

@xameer for natural numbers (that is integers which are non negative) the natural order is “less than” - a is less than b if there is another natural number c such that a plus c is b. Under that ordering, the positive integers are well-ordered. Natural is a sort of common undefined mathism meaning the obvious one. 1 < 2 is the obvious order or the natural order for positive integers, also called the natural numbers.

@jayalane fair enough so entire context was that within N ie {1,2} is guaranteed to be a subset of {1,3}